The Tanglenomicon

Zachary Bryhtan, Nicholas Connolly, Isabel Darcy, Ethan Rooke, Joseph Starr*

Mathematics Department at The University of Iowa

Knots

“A knot is a smooth embedding of a circle $S^1$ into Euclidean 3-dimensional space $\R^3$ (or the 3-dimensional sphere $S^3$ ).”

$\quad$
$\quad$
$\quad$

Jablan, S., & Sazdanović, R. (2007). Linknot. In Series on Knots and Everything. WORLD SCIENTIFIC. https://doi.org/10.1142/6623

https://www.knotplot.com/

Knot Tables

Lord Kelvin’s vortex theory of the atom. Atoms are knotted vortices in the æther.
  • 1860’s Tait computes knots up to 7 crossing
    • 15 knots
  • 1870’s Tait, Kirkman, and Little compute knots up to 10 crossing
    • Takes about 25 years
    • 250 knots
  • 1960’s Conway computes knots up to 11 crossings
    • “A few hours”
    • 802 knots
  • 1980’s Dowker and Thistlethwaite compute up to 13 crossings
    • First using a computer
    • 12,966
  • 1990’s Hoste, Thistlethwaite, and Weeks compute up to 16 crossings
    • Computer runtime on the order of weeks
    • 1,701,936
  • 2020’s Burton computes up to 19 crossings
    • 350 Million

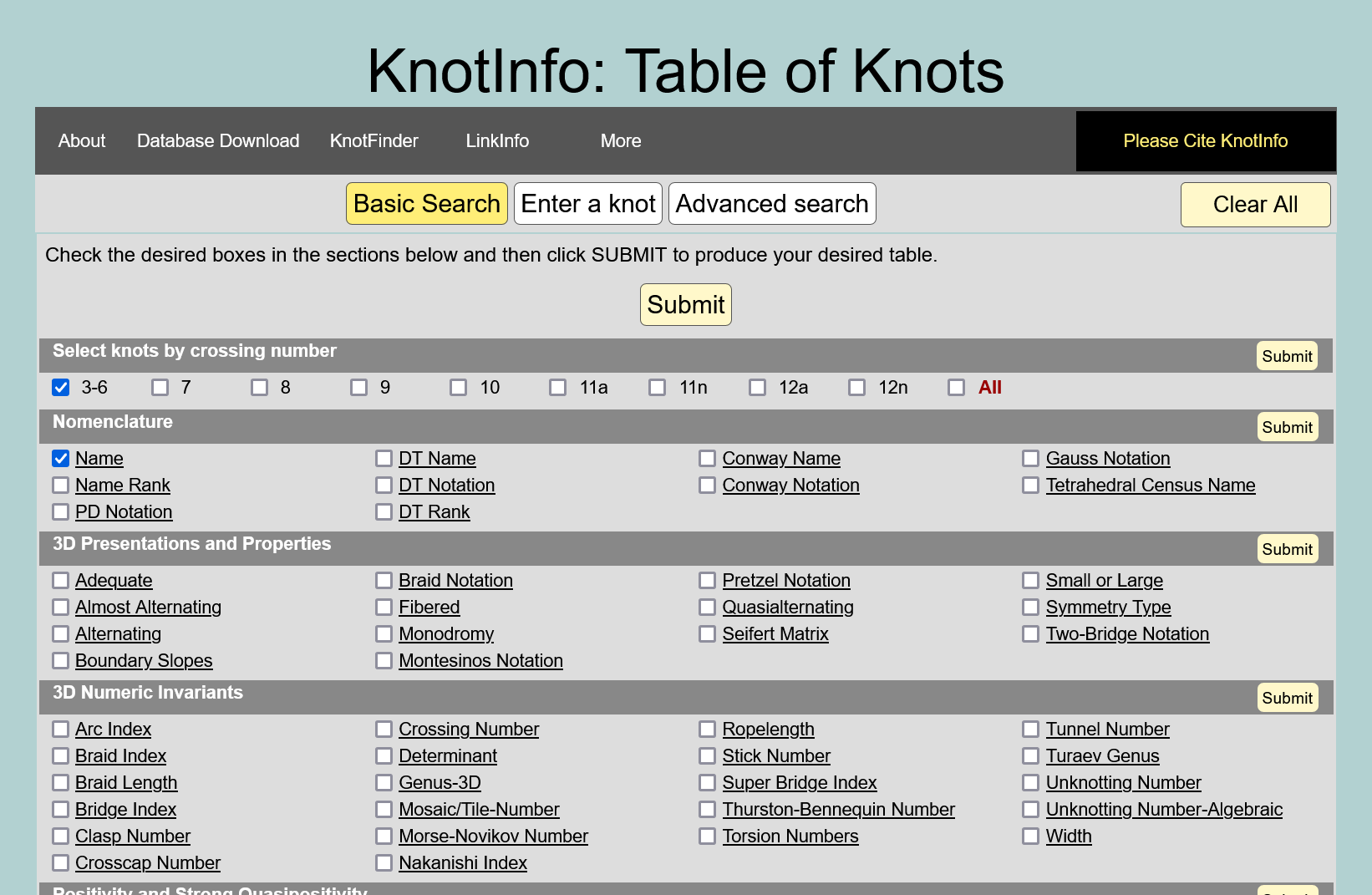
KnotInfo

Conway

How did Conway compute 25 years of work in "a few hours"?

Tangles

“We define a tangle as a portion of a knot diagram from which there emerge just 4 arcs pointing in the compass directions NW, NE, SW, SE.”

Conway, J.H. “An Enumeration of Knots and Links, and Some of Their Algebraic Properties.” In Computational Problems in Abstract Algebra, 329-58. Elsevier, 1970. https://doi.org/10.1016/B978-0-08-012975-4.50034-5

$\quad$
$\quad$
NWNESWSE
$\quad$
$\quad$
$\quad$

Basic Operations

Operation $+$

$+$
$=$
$=$
$=$
$2$

Operation $\vee$

$\vee$
$=$
$=$
$=$
$\frac{1}{2}$

The Tanglenomicon

Building up

$\ $
$\ $
$\ $
$\ $

Where we are

Rational Tangles

$\ $
$\begin{aligned}\to&\ \LP 3 \vee \frac{1}{2}\RP + 2\\&\\ \to&\ [3\ 2\ 2]\end{aligned}$

Where we’re going

Montesinos

$+$
$=$
$$\ =\ $$
$$=[3\ 2\ 2] + [3\ 2\ 2]$$

Generalized Montesinos 

<img class="centerImg" style=" width:35rem;   " src=" /presentations/lightning/GenMont.svg"/>

Operation $\circ$

$\ $
$= \color{var(--r-Purple)}([1\ 2\ 0] + [1\ 2\ 0] + [1\ 1\ 0]) \color{var(--r-Foreground)}\circ \color{var(--r-Red)}[1\ 2]$

Into the future

Algebraic

All possible tangles made from $+$ and $\vee$

Algebraic
A vertical sum of two Montesinos tangles.

Non-algebraic/Polygonal

4-valent planar graphs 

<img class="centerImg" style=" width:15rem;   " src=" /presentations/general/1star.svg"/>

<img class="centerImg" style=" width:30rem;   " src=" /presentations/general/6star.svg"/>

4-valent planar graph insertions

Some useful links

Personal Site

joe-starr.com

MGB

mathgradboard.com

KnotPlot

knotplot.com

Knot Info

knotinfo.math.indiana.edu

Illustrating Mathematics Discord

discord.gg/jedHjNgZn

Seminars

  • Topology Reading Seminar
    • T 2pm-3pm
    • 221 MLH
  • Knot, Tangles, and Computers
    • Th 11:30am - 12:30pm
  • Topology Research Seminar
    • Th 2pm-3pm
    • 221 MLH
  • Topological Data Visualization
    • F 3:30pm - 4:30pm
    • B13 MLH

Questions?

Sources

  1. Dror Bar-Natan The Most Important Missing Infrastructure Project in Knot Theory
  2. Kauffman, L. H., and S. Lambropoulou. “From Tangle Fractions to DNA.” In Topology in Molecular Biology, edited by Michail Ilych Monastyrsky, 69-110. Biological and Medical Physics, Biomedical Engineering. Berlin, Heidelberg: Springer Berlin Heidelberg, 2007. https://doi.org/10.1007/978-3-540-49858-2_5.
  3. Moon, Hyeyoung, and Isabel K. Darcy. “Tangle Equations Involving Montesinos Links.” Journal of Knot Theory and Its Ramifications 30, no. 08 (July 2021): 2150060. https://doi.org/10.1142/S0218216521500607.
  4. Conway, J.H. “An Enumeration of Knots and Links, and Some of Their Algebraic Properties.” In Computational Problems in Abstract Algebra, 329-58. Elsevier, 1970. https://doi.org/10.1016/B978-0-08-012975-4.50034-5.
  5. Louis H. Kauffman and Sofia Lambropoulou. Classifying and applying rational knots and rational tangles. In DeTurck, editor, Contemporary Mathematics, volume 304, pages 223-259, 2001
  6. Alain Caudron. Classification des nœuds et des enlacements, volume 4 of Publications Math ́ematiques d’Orsay 82 [Mathematical Publications of Orsay 82]. Universit ́e de ParisSud, D ́epartement de Mathe ́matique, Orsay, 1982.
  7. Robert Glenn Scharein. Interactive topological drawing. ProQuest LLC, Ann Arbor, MI, 1998. Thesis (Ph.D. The University of British Columbia (Canada). URL: https://www.knotplot.com/.
  8. Jablan, S., & Sazdanović, R. (2007). Linknot. In Series on Knots and Everything. WORLD SCIENTIFIC. https://doi.org/10.1142/6623
  9. Dowker, C. H., & Thistlethwaite, M. B. (1983). Classification of knot projections. In Topology and its Applications (Vol. 16, Issue 1, pp. 19-31). Elsevier BV. https://doi.org/10.1016/0166-8641(83)90004-4
  10. Hoste, J., Thistlethwaite, M., & Weeks, J. (1998). The first 1,701,936 knots. In The Mathematical Intelligencer (Vol. 20, Issue 4, pp. 33-48). Springer Science and Business Media LLC. https://doi.org/10.1007/bf03025227
  11. Burton, B. A. (2020). The Next 350 Million Knots. Schloss Dagstuhl - Leibniz-Zentrum Für Informatik. https://doi.org/10.4230/LIPICS.SOCG.2020.25
  12. C. Livingston and A. H. Moore, KnotInfo: Table of Knot Invariants, knotinfo.math.indiana.edu, today’s date (eg. August 24, 2023).